Answers to Quiz 1.
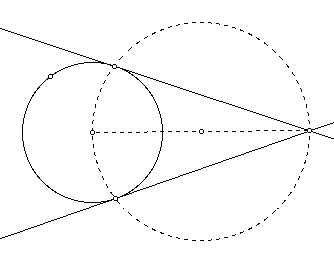
Problem 1. Tangent Construction (20 points).
This construction is described in the &B Construction Chapter and the GTC Carpenter Chapter.
Construct the midpoint of AB (by the perpendicular bisector of AB) and then construct the circle through B (or A) with center at the midpoint. The points of intersection of the two circles are the points on tangency.

Problem 2. Right triangle problem (20 points).
Prove:
Let ABC be a right triangle, with angle A the right angle. Prove that point M, the midpoint of the hypotenuse of ABC, is equidistant from the 3 vertices, A, B, C.
Proof #1
Let N be the midpoint of AB.
Construct the perpendicular bisector of AB.
This line intersects the hypotenuse BC at a point T. (The lines are not parallel since angle B is not a right angle.)
T is the midpoint M of BC.
We observe that triangle ABC is similar to triangle NBT by AA, for each has a right angle (angle BAC = angle BNT) and also the shared angle B. The ratio of similitude is BN/BA = 1/2 by definition of the midpoint.
T is the point M.
BT/BC = 1/2 by corresponding parts. Thus BT = (1/2)BC = BM, by definition of the midpoint. Since both T and M are on segment BC, T=M.
The line MN is the perpendicular bisector of AB.
Two points determine a line and now we know that both M and N are on the line.
Triangle ANM is congruent to triangle BNM.
This is true by SAS. We know that angles ANM and BNM are both right angles; NM = NM, and also AN=BN by definition of the midpoint.
AM = BM = CM
The first equality is true by corresponding parts of the triangles above and the second was given.
QED.
Proof #2
Let N be the midpoint of AB. Construct segment MN.
Triangle ABC is similar to triangle NBM.
Since NB = (1/2) AB and MB = (1/2)CB, triangle ABC is similar to triangle NBM by SAS (or Thales), since both sides have a common angle ABC = NBM.
Segment NM is perpendicular to AB.
This is true by corresponding angles: angle MNB = angle CAB = 90 degrees.
Now we can finish as before:
Triangle ANM is congruent to triangle BNM.
This is true by SAS. We know that angles ANM and BNM are both right angles; NM = NM, and also AN=BN by definition of the midpoint.
AM = BM = CM
Just as in Proof #1.
QED.
Proof #3
Let p be the parallel to BC through M. Let p intersect AB at N.
Claim: N is the midpoint of AB.
To prove this, we observe that
Triangle ABC is similar to triangle NBM
Since angle NMB = angle ACB (corresponding angles of transversal to parallel lines) and the common angle ABC = NBM, the triangles are similar by AA. Since MB = (1/2)CB, the ratio of similitude is 1/2, so NB = (1/2) AB by corresponding parts of similar triangles.
Triangle ANM is congruent to triangle BNM.
Since NB = (1/2) AB, AN also = (1/2)A; and N is the midpoint of AB, with NB = NA. . We know that angles ANM and BNM are both right angles by corresponding parts in similar triangles. Also, NM = NM. Thus the triangles are congruent by SAS.
AM = BM = CM
Just as in Proof #1.
QED.