Angle-Side-Angle

Using words:

If two angle in one triangle are congruent to two angles of a second triangle, and also if the included sides are congruent, then the triangles are congruent.

Using labels:

If in triangles ABC and DEF, angle A = angle D, angle B = angle E, and AB = DE, then triangle ABC is congruent to triangle DEF.

Proof:

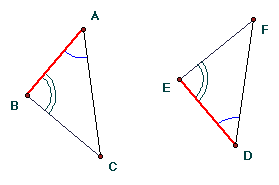
In the figure, the known congruent segments and angles in triangles ABC and DEF are color-coded.

Construct a point F' on ray AC so that AF' = DF. Angle BAF' = angle BAC and this = angle EDF and AB = DE (given), so triangle DEF = triangle ABF'.

There are two possibilities for point F': F' is the same as point C or it is not.

  • If F' is not C, then F' is not on ray BC, since line AC and ray BC only intersect at C. Thus the angle ABF' is not = angle ABC. But this is a contradiction, since angle ABF' = angle DEF (because triangle DEF = triangle ABF') and angle DEF = angle ABC (given). So this case cannot occur.
  • So it must be true that F' = C. Then triangle ABC = triangle ABF' = triangle DEF.

QED