Transversals and Angles Once and For All

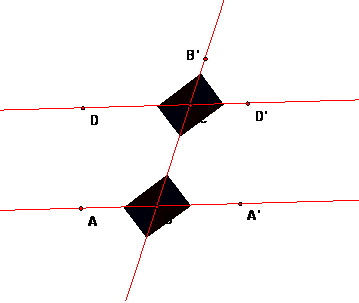
In BG, it is proved that, given two lines AA' and DD' and a transversal, the two lines are parallel if and only if the alternate interior angles formed with the transversal are equal.

This is true, but as you know, it is not the whole story, and there has been a lot of detailed proofs turned in that tell the variants this story.  It is now time to declare these additional relationships proved and "quotable."

Specifically, suppose there are lines AA' and DD' and a transversal B'C' that intersects AA' at B and DD' at C, as shown.  Assume that A and D are on the same side of the transversal and A' and D' are on the other side.  Also assume that the points on the transversal are in the order C'BCB' as shown.  The 8 angles are shown in red and blue.

Now there are two theorems that are true, that follow from the theorem in BG and from vertical angles and angle addition:

1. If AA' and DD' are parallel, all the red angles are congruent to each other and all the blue angles are congruent to each other: furthermore any red angle and any blue angle are supplementary. This is the Euclidean Parallel Postulate (plus a couple of lemmas).

2. If any red angle with vertex B is congruent to any red angle with vertex C -- or equivalently if any red angle with vertex B is supplementary to a blue angle with vertex C – then the lines AA' and DD' are parallel. 

What to say when you prove a theorem.

• Make very clear not only what the lines AA' and DD' are, but also what the transversal is.
• Then say something like this: angle X = angle Y, so "by the theorems on transversals and parallels" the lines are parallel.  Or say "since the lines are parallel, angles X and Y are congruent."

More Detail:  If you want to spell it out more using terminology, then you can quote the theorem for various combinations without proving these relationships every time.

There are more variations, but I am not sure that I have ever seen terminology used for the exterior angles.